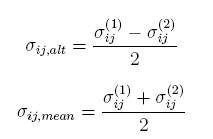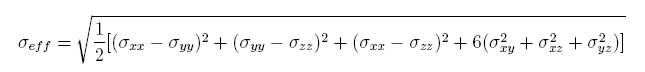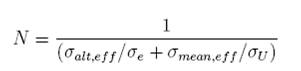Finite Element Analysis (FEA) is routinely used to perform fatigue analyses of cardiovascular stents. For the case of balloon expandable stents, this means modeling the crimping of the stent onto the delivery balloon, the expansion and recoil of the stent as would occur during deployment and finally the simulation of fatigue deformations. Fatigue deformations typically involve uniform radial pulsatile loading and/or bending of the stent between two different radii of curvature. A safety factor is determined by computing the alternating and mean stresses for the given cyclic loading conditions and comparing them to allowable material limits using a Goodman-type approach.
A simple method for determining the safety factor is to compute the alternating and mean stresses from the principle stress components for the minimum and maximum extremes of the cyclic loading conditions. The ratios of the alternating stress and endurance limit and the mean stress and the ultimate strength are combined and equated to the reciprocal of the safety factor, N.

This equation is evaluated for each integration point in the model. But as was discussed in our most recent ASTM F04.30.06 Fatigue to Fracture task group, this approach has several shortcomings.
An alternative approach is to use the individual stress components with respect to the global coordinate system for the two fatigue conditions and compute the individual alternating and mean stress components according to
These individual alternating and mean stress components are used to compute an effective alternating and an effective mean stress using
Subsequently, the effective alternating and mean stress values are used to compute a safety factor according to
An alternative approach would be to use the three principle stress components and compute the three alternating and mean principle stresses and then compute the effective stress according to the Gough–Pollard model; however, this approach still does not account for the effect of rotations in stress space on the mean and effective stress.
The exact approach would be to compute the alternating and mean stress components from the six stress components according to an element local coordinate system. However, in the case of the small fatigue motion associated with a cardiovascular stent, the element rotations between the two fatigue locations are small and therefore the effects are assumed to be negligible.
But now let’s put all of this in perspective.
For balloon expandable stents: The maximum stresses in a stent subjected to characteristic radial fatigue deformations typically occurs near the inner apexes of struts and even under the largest of deformations, there is often very little rotation. Therefore, it is anticipated that the effort to account for the individual stress components will not make a significant difference. In fact, for a typical coronary stent, the difference between the standard approach and an approached based on an updated coordinate frame as described above will at most be on the order of a few percent.
For self expanding Nitinol stents, the situation is a bit different because there can exist a shear strain component to the strain tensor which can reverse orientation and thus it’s effect can be cancelled by neglecting to look at the full strain tensor on an individual component level. Significant differences in predicted alternating strains can be obtained for load-controlled scenarios where the stent undergoes cyclic loading/unloading
In both of these cases, however, we need to keep things in persepctive. All this effort in considering the multiaxial state of stress may end up secondary when we consider that we generally only have UNIAXIAL material limit data available. The extension from uniaxial observations to multiaxial predictions is still the subject of much debate even for what are considered to be relatively well understood concepts such as plasticity. This point is important to keep in mind as we move forward with our Fatigue to Fracture initiative. Whether we take the extra effort to compute fatigue stresses according to the method described here, or by other approaches, we are still basing our predictions on material information and fatigue theories that come with their own inherent limitations. The best we can do is be consistent, conservative and use our observations and experience to improve our knowledge and condfidence in making lifetime predictions.